
Volume rectangular prism formula cracker#
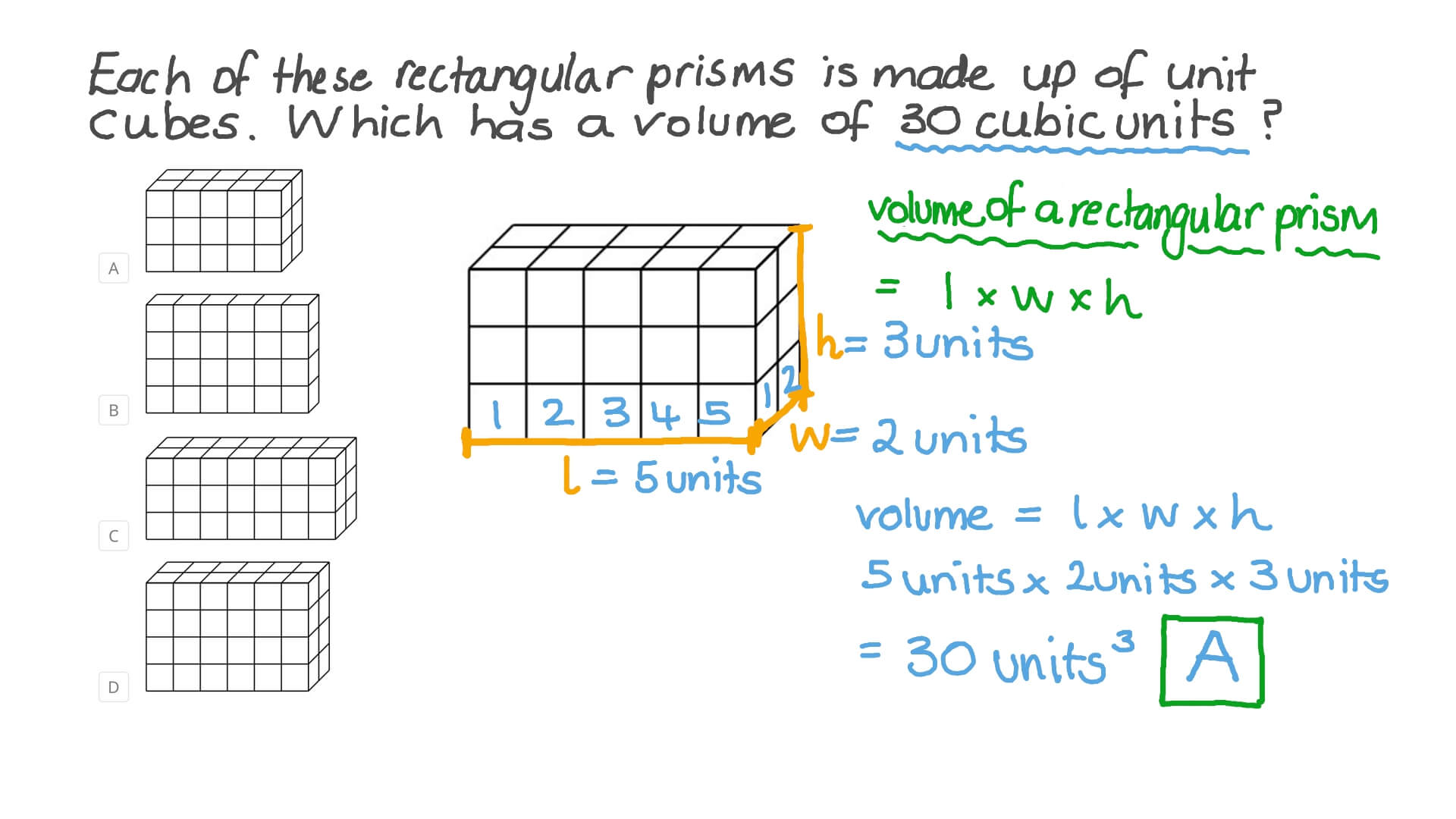
Instructional Task 1 ( MTR.2.1) The Great Graham Cracker Company is looking for a new package design for next year’s boxes. For the second figure, students would find area and use the formula b × h with b = 16. For the first figure, students would use volume and the formulaī × h with B = 16 × 4. Students then highlight the measurements used for the base in the formula. The teacher provides students with a set of models like the one shown below asking which image they would use the area formula for and which image they would use the volume formula for. Then, they use the base to calculate the area or volume.
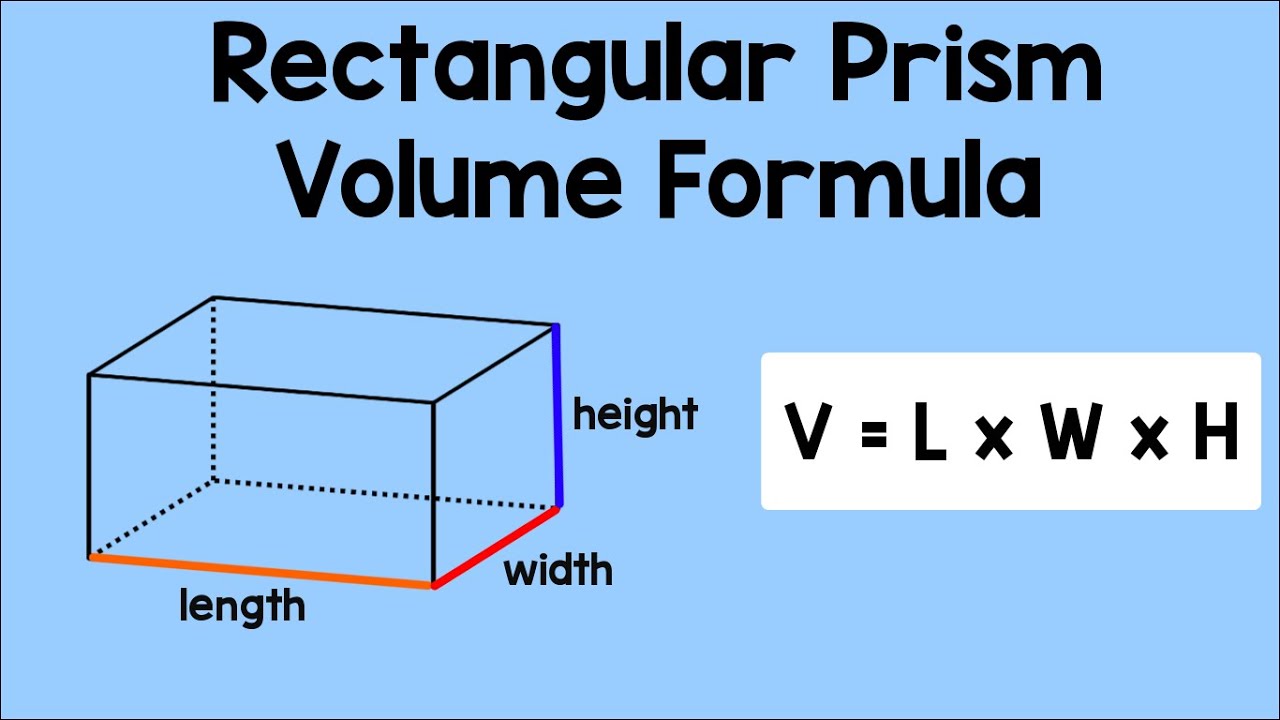
Encourage them to estimate reasonable solutions before calculating and justify their solutions after. Students may make computational errors when calculating volume.When building understanding of the volume formula for right rectangular prisms, teachers and students should include a visual model to justify their calculations. Students may confuse the difference between b in the area formula A= b X h and B in the volume formula V = B × h.This will help students understand that when calculating the volume of a rectangular prism, the three dimensions are multiplied together and that the order of factors does not matter (commutative property of multiplication). When students use a multiplication formula, it is important for them to see that it is a matter of choice which dimensions of rectangular prisms are named length, width and height.If students understand conceptually what the formulas mean, they are more likely to use them effectively and efficiently ( MTR.5.1).
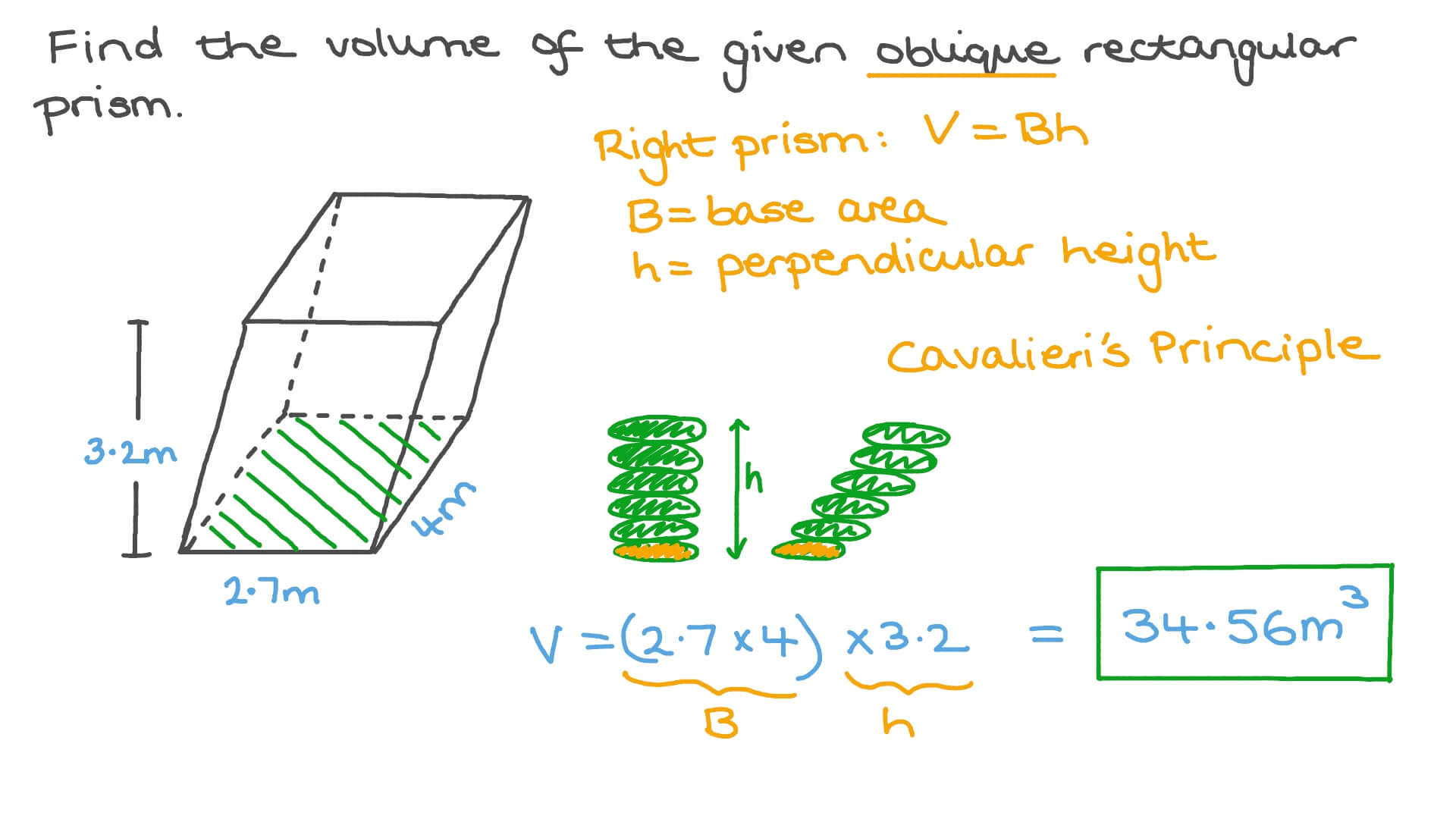
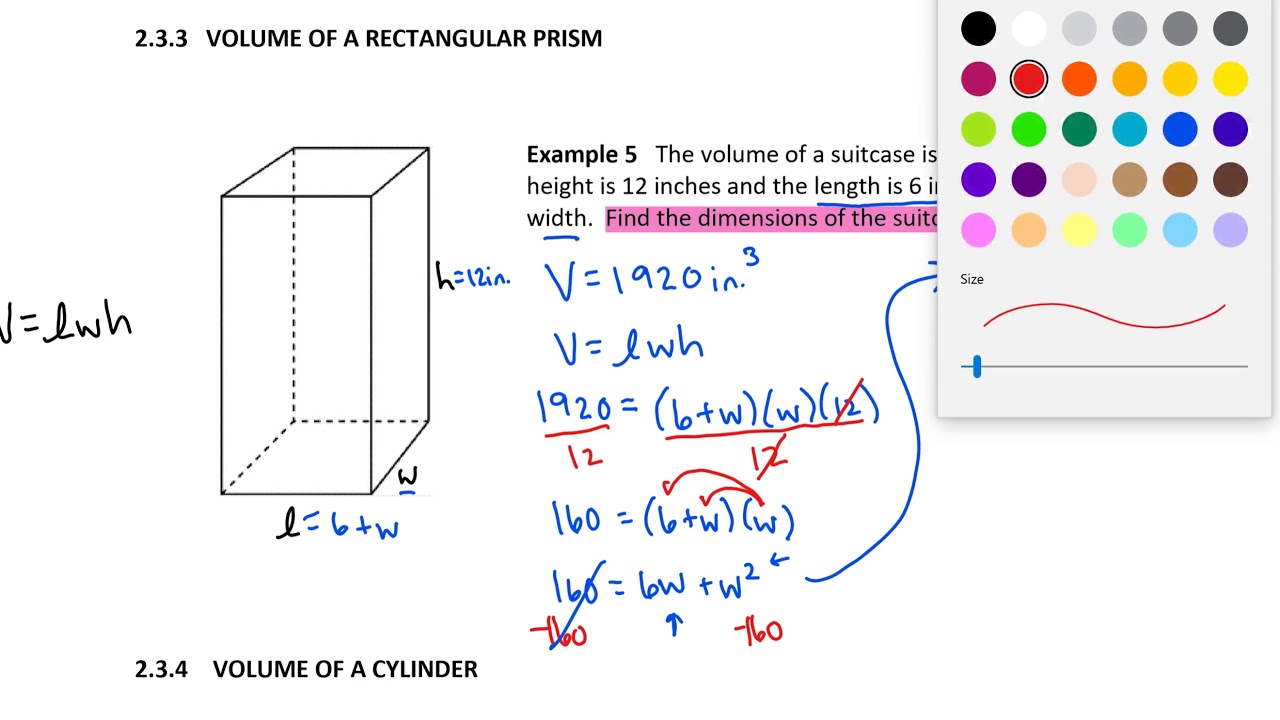
Having students explore how volume is calculated helps students see the patterns and develop a multiplication formula that will help them make sense of the two most common volume formulas, V = B × h (where B represents the area of the rectangular prism’s base) and V = l × w × h.From there, the third dimension (height) of the prism is calculated by the number of layers stacked atop one another.
The bottom layer of the prism is packed with a number of rows with a number of cubes in each, like area of a rectangle is calculated with unit squares. Instruction should begin by connecting the measurement of a right rectangular prism to the calculation of a rectangle’s area.Instruction should make connections between the exploration expected of MA.5.GR.3.1 and what is happening mathematically when calculating volume ( MTR.2.1).For volume, side lengths are limited to whole numbers in grade 5, and problems extend to fraction and decimal side lengths in grade 6 ( MA.6.GR.2.3). Students have developed experience with area since grade 3 ( MA.3.GR.2.2). The purpose of this benchmark is for students to make connections between packing a right rectangular prism with unit cubes to determine its volume and developing and applying a multiplication formula to calculate it more efficiently.Two angles whose measurements add up to 90º.Ī solid figure with a single circular base and a round, smooth face that diminishes to a single point.Īngles of separate figures that are in the same position within each figure.Connecting Benchmarks/Horizontal Alignment The distance around a circle, calculated by the formula \(C = \pi d\). The amount of space inside a two-dimensional shape, measured in square units. ^3\).Ī figure formed by the joining of two rays with a common endpoint.


 0 kommentar(er)
0 kommentar(er)
